Young’s experiment

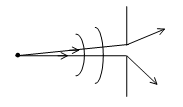
Thomas Young first demonstrated interference from light waves with a double slit in 1801. The schematic diagram for this experiment is shown in below

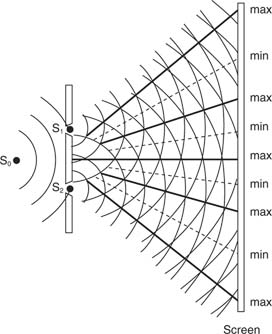
The single light source is located at S 0, and the light goes through two very narrow openings at S 1 and S 2. (A single light source is necessary because the light waves must have identical frequency and phase. The light beam is also considered to be of one color. Each of the slits is act as a source for circular expanding waves. The points of intersection of two crests, one from each slit, are points of constructive interference. The point of intersection of a crest from one slit and a trough from the other slit is a point of destructive interference. Therefore, the interference pattern called fringes, consisting of alternating light and dark bars, will be seen on the screen.

 

To better understand see the below picture for how these points are formed. It illustrates the rays coming through two slits that are directed to the point P on the screen.

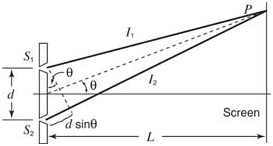
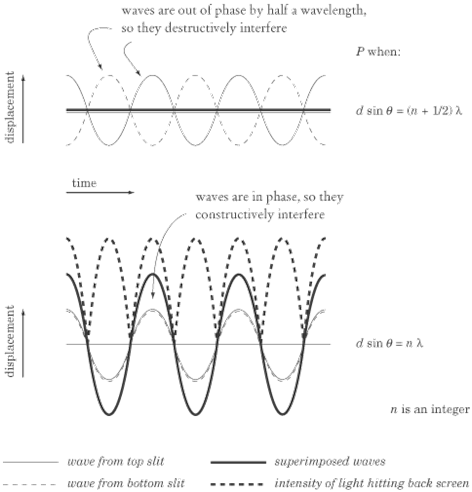
As a result, the two beams of light arrive at P out of phase by d sin . If d sin  = (n + 1/2) , where n is an integer, then the two waves are half a wavelength out of phase and will destructively interfere. In other words, the two waves cancel each other out, so no light hits the screen at P. These points are called the minima of the pattern.

On the other hand, if d sin  = n , then the two waves are in phase and constructively interfere, so the most light hits the screen at these points. Accordingly, these points are called the maxima of the pattern.

Because the far screen alternates between patches of constructive and destructive interference, the light shining through the two slits will look something like this

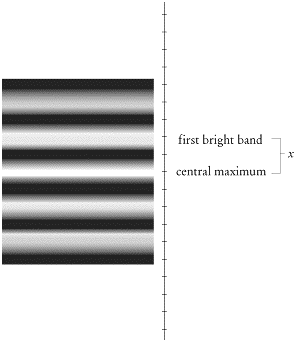
Note that the pattern is brightest in the middle, where   = 0. This point is called the central maximum. If you encounter a question regarding double-slit refraction on the test, you’ll most likely be asked to calculate the distance x between the central maximum and the next band of light on the screen. This distance, for reasons too involved to address here, is a function of the light’s wavelength ( ), the distance between the two slits (d), and the distance between the two screens (L):

The difference in path length of the two rays is given by d sin θ = l ₂ = l₁. If the path difference is a whole number of wavelengths, then constructive interference takes place. If the paths differ by a half number of wave lengths, destructive interference occurs. Using n to represent any integer, the two cases may be written

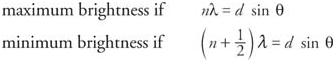
Fringe Width:- It is the distance between two consecutive bright and dark fringes.

β = λD/d

Displacement of fringes due to the introduction of a thin transparent medium:-

(a) Shift for a particular order of fringes:-

y = (β/λ) (µ-1)t

(b) Shift across a particular point of observation:-

µ = (mλ/t) +1

Lloyd’s single mirror:-

?λ = β .2a/D

Power of lens:- P= 100/f

Magnifying power or magnification of a simple microscope:-M= 1+(D/f)

Magnifying power or magnification of a compound microscope:-

M= L/f0 (1+D/fe)

Here, f0 is the focal length of the objective, fe is the focal length of the eyepiece and L is the length of the microscope tube.

Magnification of astronomical telescope in normal adjustment:-

M = f0/fe

Magnification of astronomical telescope, when the final image is formed at the distance of distinct vision:-

M = (f0/fe) [(fe+D)/D]

Magnifying power M of Galileo’s telescope:-

M = focal length of objective/focal length of eye lens = F/f

Diffraction:- Diffraction is the bending  or spreading of waves that encounter an object ( a barrier or an opening) in their path.

(a) In Fresnel class of diffraction, the source and/or screen are at a finite distance from the aperture.

(b) In Fraunhofer class of diffraction, the source and screen are at infinite distance from the diffracting aperture. Fraunhofer is a special case of Fresnel diffraction.

If Im represents the intensity at O, its value at P is

Iθ = Im (sinα/α)2

Here, α = ?/2 = πa sinθ/λ

A minimum occurs when, sin α = 0 and α is not equal to zero.

so  α = nπ,       n = 1, 2, 3…

So, πa sinθ/λ = nπ

Or, a sinθ = nλ

Angular width of central maxima of diffraction pattern = 2θ1 = 2 sin-1(λ/a)

[ θ1 gives the angular position of first minima]